Schreiber Proper Orbifold Cohomotopy for M-Theory

Skip the Navigation Links | Home Page | All Pages | Latest Revisions | Authors |


A talk that I have given:

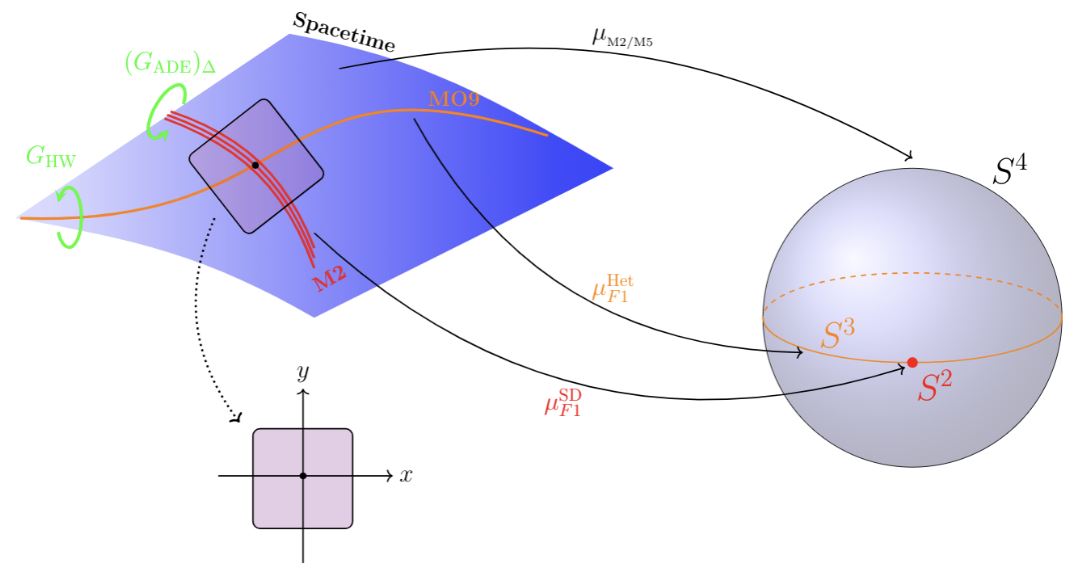
Abstract. M-theory folklore has it that all quasi-realistic QCD-like quantum physics is realized (and thereby non-perturbatively completed) at or inside super-spacetime orbi-singularities of intersecting black M5/M2-branes, whose higher magnetic flux threads an ambient smooth G-structured bulk super-spacetime subject to a long list of subtle differential- and algebro-topological constraints (such as shifted C-field flux quantization, C-field tadpole/RR-field tadpole cancellation, GS-anomaly cancellation, etc.).

Despite an intriguing web of plausibility arguments and consistency checks that have been amassed since this picture emerged in the “second superstring revolution”, it has remained unclear, and largely unexplored, what any of this really means mathematically – notably what putative M-theory on fluxed and G-structured super-orbifold spacetimes actually predicts, with any certainty, for observable physics – be it in its application to confined hadronic matter (holographic QCD), or to quantum-supreme solid states (holographic CMT), not to speak of the more traditional but less tangible application to quantum gravity.

The issue with the mathematical foundations of M-theory (formerly known as string theory) had been prophesized early on (E. Witten 1988, in Davis & Brown, CUP 1988, p. 95, 102): “String theory at its finest should be a new branch of geometry …\ldots developed in the twenty-first or twenty-second century …\ldots that fell by chance into the twentieth century”.

With the 21st century now well under way, this talk surveys a new branch of mathematical geometry which has emerged over the last years, in joint work with Hisham Sati, and which provably exhibits a fair number of the subtle phenomena expected in M-theory on fluxed orbifold spacetimes:

equivariant Cartan cohomotopy, from https://ncatlab.org/schreiber/show/Equivariant+homotopy+and+super+M-branes

This “differential cohomotopy in a singular-cohesive homotopy-topos” (arXiv:2008.01101) operates close to novel homotopy-theoretic foundations for mathematics itself (modal/cohesive homotopy type theory) and provides a rigorous but charmingly intuitive axiomatics of geometric qualities that transparently control elaborate higher geometric entities such as the twisted equivariant differential generalized cohomology of G-structured super-orbifolds which should give mathematical meaning to quantized M-brane charges and C-field fluxes.

In this talk I will try to give some idea of the basics and of some applications and results, following the references listed below.

Evidence for the natural “Hypothesis H” that this new geometry is part of the missing mathematical foundations of M-theory is further discussed in the lecture by Hisham Sati in the school the week before this conference.


Related talks:



For more

see the pointers at Hypothesis H.


Last revised on December 8, 2021 at 16:35:16. See the history of this page for a list of all contributions to it.

EditDiscussPrevious revisionChanges from previous revisionHistory (17 revisions) Print Source